
The British Columbia Parliament Buildings are located in Victoria, British Columbia, Canada and serve as the seat of the Legislative Assembly of British Columbia. The main block of the Parliament Buildings combines Baroque details with Romanesque Revival rustication.
Location: Victoria, British Columbia, Canada
Image ID: 21048
Location: Victoria, British Columbia, Canada
Image ID: 21048
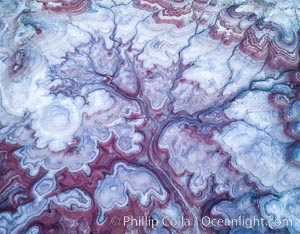
Pre-Dawn over the Tree of Eons, Utah. The Tree of Eons is a spectacular geologic sight near the San Rafael Swell. Erosion has cut a "tree" through red, blue, purple and white layers of the Chinle formation. The Tree of Eons is a superb example of dendritic erosion and to really appreciate the complex fractal-like details it must be observed from above. Photographed here in the soft, predawn light, it takes on magenta, red and purple hues just before the sun reaches it. Aerial panoramic photograph.
Location: Utah
Image ID: 38027
Location: Utah
Image ID: 38027

Starfish detail, sea star skin details, Vancouver Island, Canada.
Location: British Columbia, Canada
Image ID: 35313
Location: British Columbia, Canada
Image ID: 35313

Starfish detail, sea star skin details, Vancouver Island, Canada.
Location: British Columbia, Canada
Image ID: 35314
Location: British Columbia, Canada
Image ID: 35314

Closeup view of stony coral polyp details, Fiji.
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31567
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31567

Closeup view of stony coral polyp details, Fiji.
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31569
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31569

Starfish detail, sea star skin details, Vancouver Island, Canada.
Location: British Columbia, Canada
Image ID: 35373
Location: British Columbia, Canada
Image ID: 35373

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10368
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10368

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10369
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10369

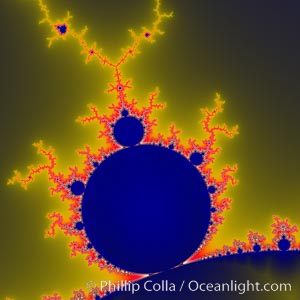
Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10375
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10375
Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10378
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10378

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10383
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10383

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10391
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10391

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10395
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10395

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18729
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18729

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18731
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18731

Fractal design. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18732
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18732

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18737
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18737
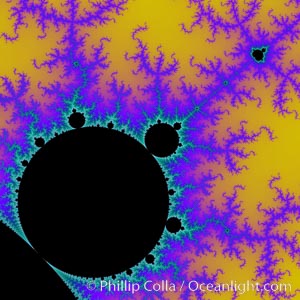
The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18739
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18739

The Tree of Eons, Utah. The Tree of Eons is a spectacular geologic sight near the San Rafael Swell in Utah. Here the Tree of Eons is seen under the direct light of midday. Erosion has cut a dendritic "tree" through red, blue, purple and white layers of the Chinle formation. The Tree of Eons is a superb example of dendritic erosion and, to really appreciate its complex fractal-like details, must be observed from above.
Location: Utah
Image ID: 38201
Location: Utah
Image ID: 38201

Ornate Ceiling Details, Vatican Museums, Vatican City.
Location: Vatican City, Rome, Italy
Image ID: 35571
Location: Vatican City, Rome, Italy
Image ID: 35571

Closeup view of stony coral polyp details, Fiji.
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31791
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31791

Closeup view of stony coral polyp details, Fiji.
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31792
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31792

Closeup view of stony coral polyp details, Fiji.
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31793
Location: Makogai Island, Lomaiviti Archipelago, Fiji
Image ID: 31793
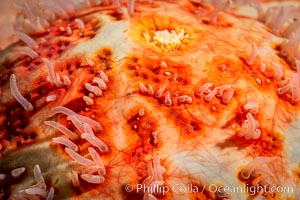
Starfish detail, sea star skin details, Vancouver Island, Canada.
Location: British Columbia, Canada
Image ID: 35443
Location: British Columbia, Canada
Image ID: 35443

Starfish detail, sea star skin details, Vancouver Island, Canada.
Location: British Columbia, Canada
Image ID: 35444
Location: British Columbia, Canada
Image ID: 35444

Sandstone details, red rocks, Valley of Fire.
Location: Valley of Fire State Park, Nevada
Image ID: 28447
Location: Valley of Fire State Park, Nevada
Image ID: 28447

Ornate Ceiling Details, Vatican Museums, Vatican City.
Location: Vatican City, Rome, Italy
Image ID: 35593
Location: Vatican City, Rome, Italy
Image ID: 35593

Ornate Ceiling Details, Vatican Museums, Vatican City.
Location: Vatican City, Rome, Italy
Image ID: 35594
Location: Vatican City, Rome, Italy
Image ID: 35594

Sandstone details, South Coyote Buttes.
Location: South Coyote Buttes, Paria Canyon-Vermilion Cliffs Wilderness, Arizona
Image ID: 26640
Location: South Coyote Buttes, Paria Canyon-Vermilion Cliffs Wilderness, Arizona
Image ID: 26640