
California sea lion on drift kelp paddy, underwater. This adult female California sea lion was hanging out underneath a paddy of drift kelp, well offshore the coastline of San Diego.
Species: California sea lion, Zalophus californianus
Location: San Diego, California
Image ID: 38543
Species: California sea lion, Zalophus californianus
Location: San Diego, California
Image ID: 38543

Aerial Photo of La Jolla coastline, showing underwater reefs and Mount Soledad.
Location: La Jolla, California
Image ID: 30676
Location: La Jolla, California
Image ID: 30676

Childrens Pool Reef Exposed at Extreme Low Tide, Casa Cove, La Jolla, California. Aerial panoramic photograph.
Location: La Jolla, California
Image ID: 40234
Panorama dimensions: 5612 x 11740
Location: La Jolla, California
Image ID: 40234
Panorama dimensions: 5612 x 11740

Children's Pool Reef Exposed at Extreme Low King Tide, La Jolla, California. Aerial panoramic photograph.
Location: La Jolla, California
Image ID: 40718
Panorama dimensions: 6238 x 11571
Location: La Jolla, California
Image ID: 40718
Panorama dimensions: 6238 x 11571

Aerial Photo of North Coronado Island, Baja California, Mexico, viewed from the southwest. The Keyhole, a spectacular narrow cut and underwater tunnel through the island, is seen at the narrow neck of the island. The San Diego and Tijuana coastline is visible in the distance.
Location: Coronado Islands (Islas Coronado), Baja California, Mexico
Image ID: 35896
Location: Coronado Islands (Islas Coronado), Baja California, Mexico
Image ID: 35896

La Jolla Shores Coastline and Scripps Pier, Blacks Beach and Torrey Pines, aerial photo, sunset.
Location: La Jolla, California
Image ID: 37485
Location: La Jolla, California
Image ID: 37485

La Jolla Shores Coastline and Scripps Pier, Blacks Beach and Torrey Pines, from Mount Soledad, sunset.
Location: La Jolla, California
Image ID: 37494
Location: La Jolla, California
Image ID: 37494

Sunset on the Del Mar Bluffs and Train Tracks, with North County coastline. The highest peaks in the distance are Santiago Peak and Modjeska Peak, the pair commonly known as Saddleback.
Location: Del Mar, California
Image ID: 37603
Panorama dimensions: 5276 x 15919
Location: Del Mar, California
Image ID: 37603
Panorama dimensions: 5276 x 15919

La Jolla Shores Coastline and Scripps Pier, aerial photo, sunset.
Location: La Jolla, California
Image ID: 36655
Panorama dimensions: 5672 x 8508
Location: La Jolla, California
Image ID: 36655
Panorama dimensions: 5672 x 8508

La Jolla Shores Coastline and Scripps Pier, Blacks Beach and Torrey Pines, aerial photo, sunset.
Location: La Jolla, California
Image ID: 36668
Panorama dimensions: 5698 x 8547
Location: La Jolla, California
Image ID: 36668
Panorama dimensions: 5698 x 8547

Camp Pendleton, Pacific coastline, north of San Diego county and the city of Oceanside. Marine Corps Base Camp Pendleton.
Location: Marine Corps Base Camp Pendleton, California
Image ID: 25980
Location: Marine Corps Base Camp Pendleton, California
Image ID: 25980

Kelp beds adorn the coastline of San Clemente Island, aerial photograph.
Species: Giant kelp, Macrocystis pyrifera
Location: San Clemente Island, California
Image ID: 25984
Species: Giant kelp, Macrocystis pyrifera
Location: San Clemente Island, California
Image ID: 25984

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10368
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10368

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10369
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10369

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10375
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10375
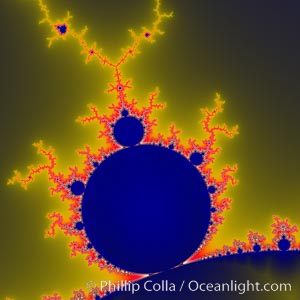
Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10378
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10378

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10383
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10383

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10391
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10391

Detail within the Mandelbrot set fractal. This detail is found by zooming in on the overall Mandelbrot set image, finding edges and buds with interesting features. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10395
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 10395

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18729
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18729

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18731
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18731

Fractal design. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18732
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18732

The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18737
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18737
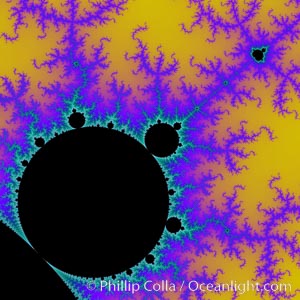
The Mandelbrot Fractal. Fractals are complex geometric shapes that exhibit repeating patterns typified by self-similarity, or the tendency for the details of a shape to appear similar to the shape itself. Often these shapes resemble patterns occurring naturally in the physical world, such as spiraling leaves, seemingly random coastlines, erosion and liquid waves. Fractals are generated through surprisingly simple underlying mathematical expressions, producing subtle and surprising patterns. The basic iterative expression for the Mandelbrot set is z = z-squared + c, operating in the complex (real, imaginary) number set.
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18739
Species: Mandelbrot fractal, Mandelbrot set
Image ID: 18739

Stone Steps and Leucdia coastline, top down view, aerial photo.
Location: Encinitas, California
Image ID: 37970
Location: Encinitas, California
Image ID: 37970

Crystal Pier and Pacific Beach coastline, Mission Bay, Point Loma and San Diego visible in the distance, aerial photo.
Location: Pacific Beach, California
Image ID: 37975
Location: Pacific Beach, California
Image ID: 37975

Sun Gold Point Reef Exposed at Extreme Low Tide, La Jolla, California.
Location: La Jolla, California
Image ID: 37984
Location: La Jolla, California
Image ID: 37984

Nicholson Point and Hospitals Beach, aerial photo, extreme low tide, La Jolla, California.
Location: La Jolla, California
Image ID: 37987
Location: La Jolla, California
Image ID: 37987

